If we were to ask a broad spectrum of poker players about the most significant differences between multi-table tournaments and cash games, we'd probably receive various answers. However, the more experienced our respondents were, the more likely we'd receive a "lack of a postflop rake" (which changes the ranges more than you might imagine!) and the "existence of ICM" as answers.
In this article, we'd like to examine what ICM is and how it should influence your decisions.
What is ICM, and why is it so important?
Independent Chip Model (ICM) evaluates the value of chip stacks in tournament poker. When you play in a cash game, any chip representing 1 dollar is worth exactly that much. After all, you can stand up from the poker table and exchange all the chips for the dollars they represent at any point in time.
As you probably know, the same "conversion rate" is not true for the tournaments. Why is that?
Imagine you play cash with nine friends on Friday night. Everyone bought in for $100, and you were so fortunate to have won all the chips there were to play for. Since no one wanted to play any longer, you'd start the weekend with the $ 1,000 in your pocket.
What if you mixed things up a little and played a 10-man $100 buy-in SNG with an a traditional payout structure (50% for 1st, 30% for 2nd and 20% for 3rd)? In that case, if you'd be lucky enough to win, you'd end up with only $500 despite achieving pretty much the same feat.
When you're playing tournament poker, your winnings are capped by the first prize amount (which, in our example, is 50% of the total prize pool), which influences the optimal strategy. While unlikely, you can theoretically win all the chips at any cash game. The same cannot be said about poker tournaments.
Subsequently, while the $1 chip is always worth $1 in the cash game, the value of the same chip will change during the different stages of the poker tournament.

Chip EV and $EV
To underline the differences in the value of chips in the two formats, we use one of two terms: Chip Expected value and Dollar Expected Value (or cEV and $EV for short). The cEV is used almost exclusively for cash games and reflects that the external circumstances in the game you are playing almost don't affect your decisions.
In other words, whenever you're facing a decision in a cash game, stacks of other players that do not participate in the hand don't matter as their sizes do not affect what you should do. The chips you win are worth roughly the same as the ones you lose, so you should make decisions based primarily on your odds of winning the hand.
In tournament poker, the value of your chip stack is affected by a few factors, e.g. how much of the total prize pool is still in play or the size of other chip stacks. At the very beginning of the tournament, the $EV of your chips is very low, but it steadily increases with the eliminations of other players.
The importance of the $EV and its distinction from the cEV is most prominent when you end up on the final table. There, the value of your chip stack and ICM strategy will differ significantly depending on the other stacks.
You have to respect the ICM pressure
What does it mean? In tournament poker, the lower your chip stack, the more valuable your chips are. It's a result of the fact that as the tournament progresses, you can earn money by folding. Whenever someone else busts, you profit, not even playing a hand or risking your chips, which is a feat impossible to achieve in cash games.
This phenomenon is most impactful during the final tables. Nowadays, an average tournament poker player is at least aware of the existence of poker ICM, but back in the day, people burned money left and right due to their lack of knowledge of how valuable their chips were.
For example, imagine you're a middle stack (around 30BB), and five other players are still in the game, all much shorter than you, except for a chip leader on the big blind. You open the CO with a pair of nines, and the action folds to the BB, who shoves against your raise. While it would be a snap call (or close to) according to cEV (i.e. without the ICM considerations), it becomes a tough decision (leaning towards the fold) when the ICM is in play!
Where does that difference come from? The presence of short stacks allows the big stack to bully the medium stacks (in our example, you) relentlessly. When you are in the middle of the pack, your tournament chip stack is valuable and fragile at the same time. You should be very risk-averse and wait for a few smaller stacks to bust out, guaranteeing yourself a pay jump or two. Quite often, it will be correct to pass on a profitable spot (in terms of earning chips) just because it's more beneficial to give shorter stacks a chance to bust.
Of course, the influence of the ICM is usually more subtle than this, but recognizing its strength is one of the most essential skills for every tournament player.
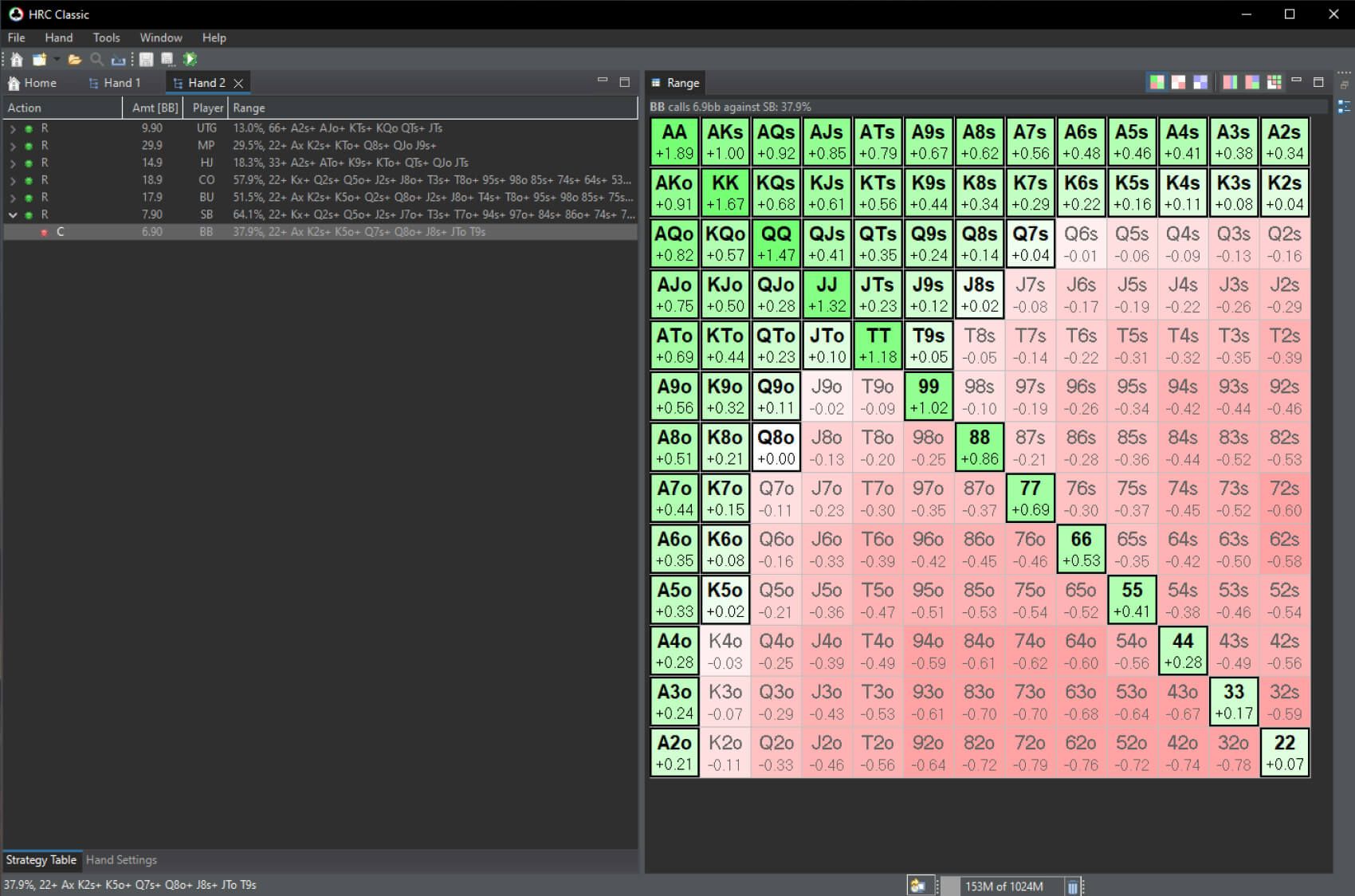
Independent Chip Model in practice
Calculating ICM influence quickly is not easy, but over time, you can familiarize yourself with it to a point at which you can roughly estimate how it should influence your strategy in real-time.
Let's picture it by extending a simple example we've used earlier. You're playing an SNG with a standard prize pool distribution (50%/30%/20%), and four people are left at the table. There are 10,000 chips in play and $1,000 to be paid out.
Let's see how the ICM value of stack sizes changes under different circumstances.
Example 1.
Let's assume the somewhat likely chip distribution, where chip stacks equal:
- You: 5000
- Player 2: 2000
- Player 3: 2000
- Player 4: 1000
In this scenario, each of the stack's worth would be (assuming the prize pool of $1000):
- You: $370.18
- Player 2: $243.3
- Player 3: $243.3
- Player 4: $141.7
As you can see, despite your stack being five times as big as the short stack, it's "worth" only 2.5 times as much in $EV! As we've said before, the short stack's value (~14% of the total prize pool) is much bigger than their share of all the chips (~10%).
Let's look at another, more extreme example with stack sizes as follows:
- You: 7000
- Player 2: 1000
- Player 3: 1000
- Player 4: 1000
In this scenario, each of the stack's worth would be:
- You: $431.67
- Players 2,3 & 4: $189.44
So, despite having a commanding chip lead (almost 70% chips in play), your stack's value is about 86% of the first place and 43% of the total prize pool. On the other hand, every other stack is worth 18% despite each having only 10% of chips in play. This time, however, we're pretty sure the metagame would be different since none of the three shorts would like to bust, given how relatively easy it is to outlast at least one other player and make it to the money.
Of course, "in real life", there are countless potential ICM scenarios. You can't master all of them, but with the help of an ICM calculator, you can get a grasp of how you should evaluate your chips $EV during the final table of a poker tournament.
You can toy around with the ICM poker calculator on the HRC site: https://www.holdemresources.net/icmcalculator
It's also worth noting that poker ICM is especially evident in satellites with multiple seats, as it doesn't matter how many chips you have as long as you finish ITM.
Quite often, the Independent Chip Model is the basis for deals in poker tournaments. How does ICM deal work? The remaining players receive the current prize, and all of their chips are then valued according to the ICM model, which dictates how the remaining prize money is allocated.
What's often surprising to many observers is that the chip lead is worth less than expected, and the opposite is true for the shorter stacks. That's how the ICM calculations work, which we've tackled in the examples above.
The ICM poker model is not flawless
While very useful, the Independent Chip Model is far from perfect. Its biggest flaw is the fact that it only takes into account the current situation on the table. When the tournament reaches the final table, each player's skill is crucial for realizing their stack's $EV — no ICM calculator accounts for that.
That's something you should always have in mind. How to include it in your poker strategy? A good rule of thumb is to be more risk-averse when you feel your opponents are worse than you and are likely to make big mistakes. At the same time, when you are playing against very tough competition, you'll likely have to make a stand and pick each, even slightly profitable, spot you'll encounter - sometimes, you won't have the luxury to not follow ICM poker.
The other thing that the Independent Chip Model omits is "the future". ICM doesn't care about what would happen in the next hand. It assumes that the game ends here and now and shows you the numbers that reflect that. While it may not always be that important, there are cases (like being on the Big Blind next hand or the level going up) that may make a difference. Also, the way ICM works does not care much about the stack distribution (i.e. who got a positional advantage) - which often heavily influences what you can and cannot expect to happen.
Lastly, whenever you consider playing for your tournament life, you must consider how the potential double-up would position you among the other players' stacks. Would it make you a chip leader who can exert additional pressure, or the playability of your stack wouldn't change much?
As you can see, there is a lot to digest regarding ICM poker. What's the best way to do so and improve your skills?
Use poker tools and focus on ICM calculators to help yourself outsmart your opponents.
ICM calculations — Which program to use?
Several programs help you understand ICM, but two are more popular than others: Holdem Resources Calculator and ICMizer. Both these poker tools work similarly and provide you with quick ICM calculations.
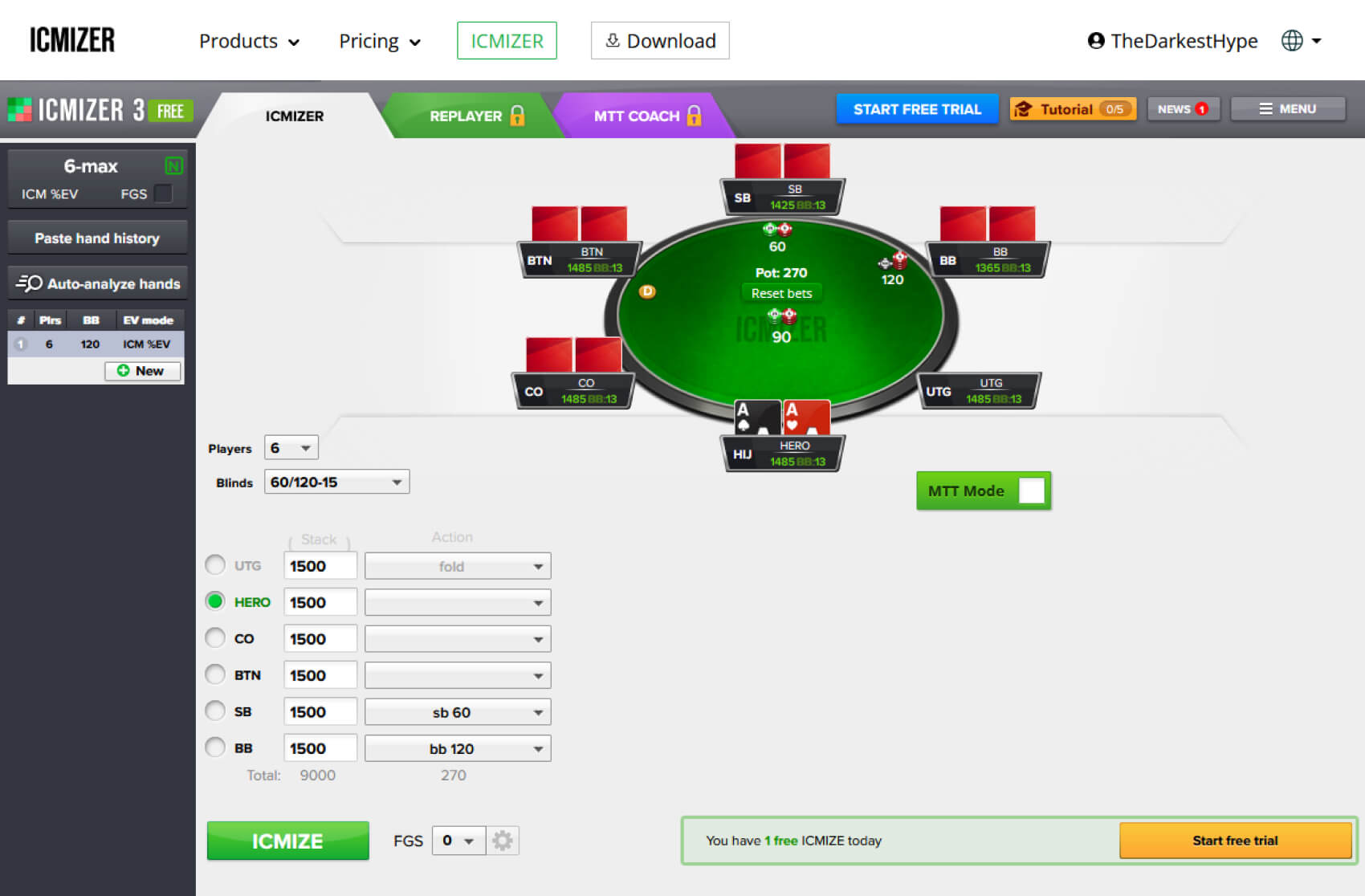
Their most important and commonly used function is providing unexploitable push/call strategies under ICM conditions. Which hand to push and which to call to avoid an "ICM suicide"? The constant development of these apps allows you to run more complex scenarios but even studying the basic ICM simulations will improve your tournament game.
In summary, if you play tournaments, there's now way around it - knowing how the Independent Chip Model works and how it should be applied is a must.
Mastering ICM poker is a key to doing well in the tournaments
But even the best ICM knowledge won't help if you're making post-flop blunders. Preflop knowledge is a solid foundation, but it is the postflop where you can generate the biggest edge against your competition.
Among the poker software that can help you improve your understanding of postflop complexity, Deepsolver is one of the best. Our tool will deliver you accurate GTO strategies quickly and effortlessly. See how it works for yourself now with the seven-day trial.
